
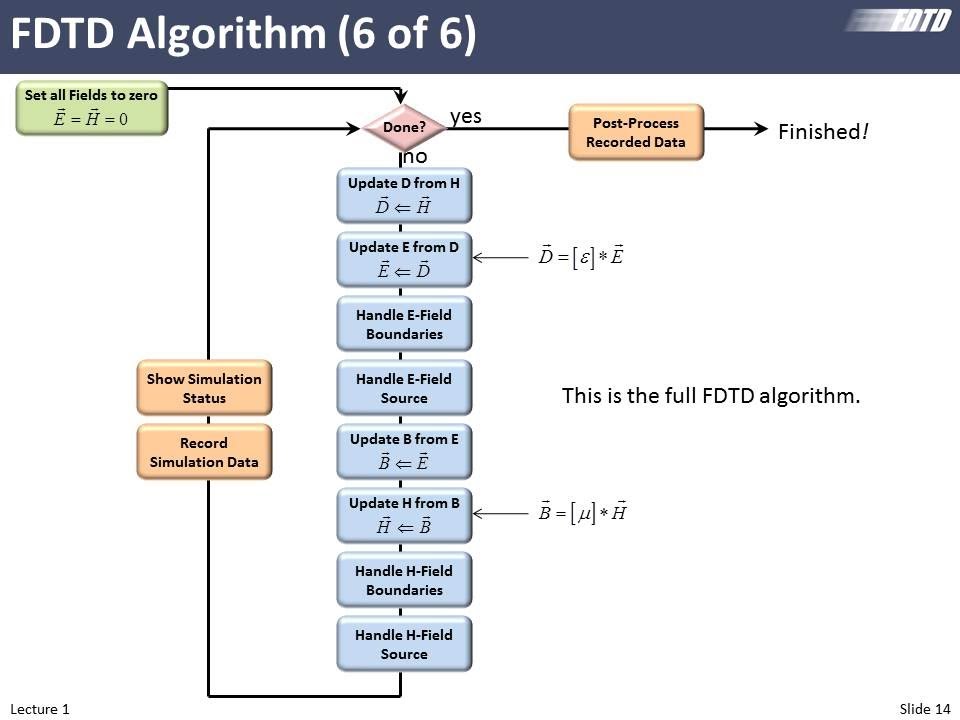
For many devices, such as SOI-based slab waveguide structures that only support two vertical modes with different polarizations, this is a very good assumption. In both cases, the key assumption is that there is negligible coupling between the different slab modes supported by the vertical waveguide structure.
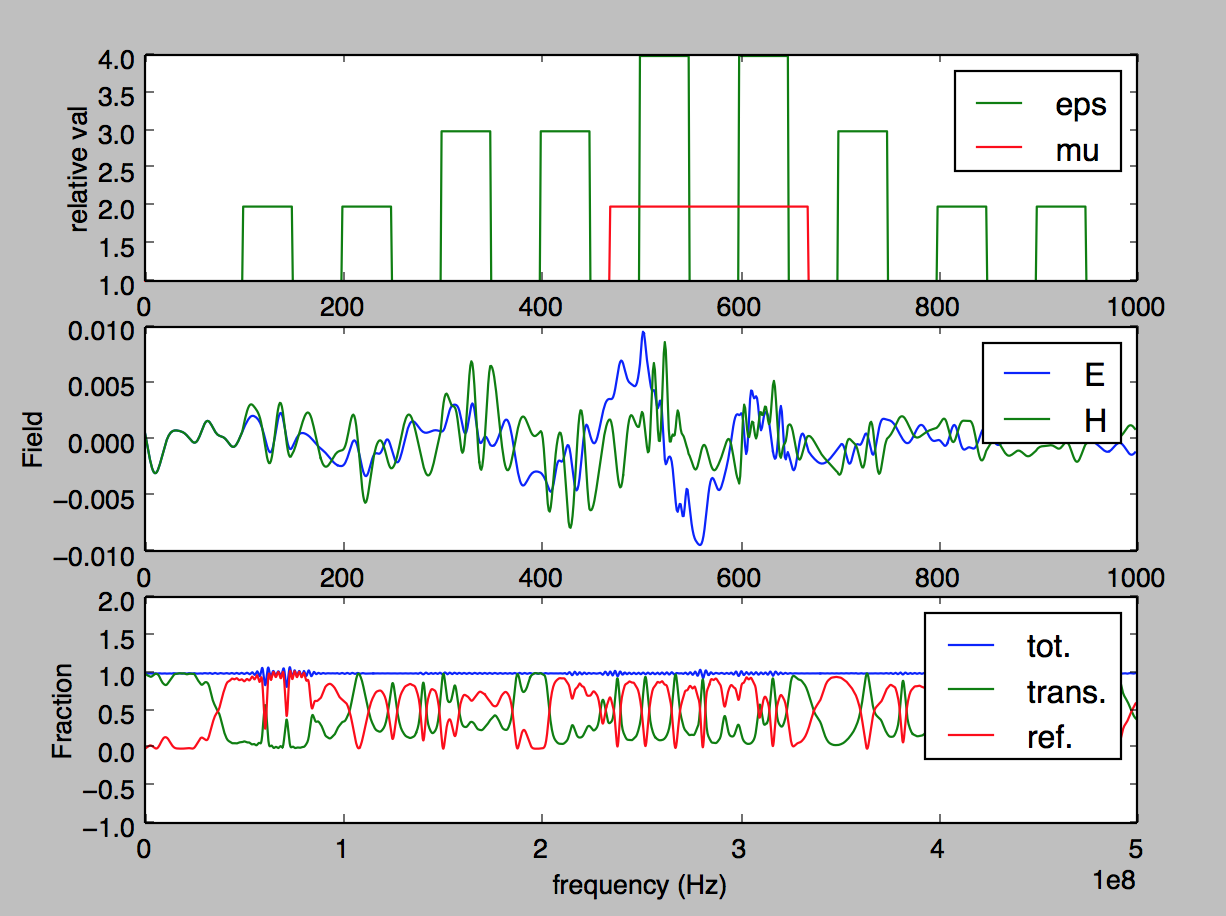
There are several alternative methods for simulating wave propagation over large distances: the well-known Beam Propagation Method (BPM) relies on a slowly varying envelope assumption and can simulate large structures quickly. However, when applied to three-dimensional structures, FDTD is highly computationally intensive, making it difficult to treat large integrated optical components efficiently. The finite-difference time-domain (FDTD) technique is one of the most versatile and accurate methods for simulating light propagation in nanoscale components. Its ability to predict the performance of optically large components more accurately than beam propagation methods, combined with its optimized computation engine, renders it a robust waveguide design environment well suited for the virtual prototyping and optimization of planar integrated optical components and circuits. Lumerical MODE Solutions’ variational FDTD (varFDTD) solver efficiently simulates the propagation of optical radiation in a wide array of guided structures, from ridge waveguide-based systems to more complex geometries such as photonic crystals. Surface.Back to White Papers Lumerical’s 2.5D variational FDTD (varFDTD) solver offers more accuracy and versatility than beam propagation method (BPM) for the virtual prototyping of planar integrated optical components The convergence ofįield values supports the hypothesis that global first-order error can beĪchieved by second-order error in bulk material with zero-order error on the Latter is stable and more accurate than other FDTD methods. al., 2011), but the symmetrized version of the Accurate treatment of theĭiscontinuities, in the limit of infinite wavelength, leads to an asymmetric, The grid cell size) when the dielectric boundaries are sharp, due to fieldĭiscontinuities at the dielectric interface. Ultimately this algorithm has first-order error (in (usually for \epsilon significantly greater than 10) with either isotropic orĪnisotropic dielectrics. al., 2009),Īnd it remedies a defect that causes instability with high dielectric contrast This algorithm is in many cases more accurate than
#Fdtd algorithm pdf
Cary (1 and 2) ((1) University of Colorado, Boulder, CO, (2) Tech-X Corporation, Boulder, CO) Download PDF Abstract: A more accurate, stable, finite-difference time-domain (FDTD) algorithm isĭeveloped for simulating Maxwell's equations with isotropic or anisotropicĭielectric materials.


 0 kommentar(er)
0 kommentar(er)
